Caps: Challenging the Conventional Wisdom that Caps are Too Expensive
Publication: AFP Exchange

In my last Risk Column in the July/August issue of Exchange, “Comparing Apples and Oranges (and Pears),” I presented a methodology for comparing swaps, caps, and swaptions for companies interested in hedging their exposure to variable interest rates on their bank loans or other variable rate borrowings. Each of these three alternative hedging instruments has a distinct economic objective, so comparing them is tricky. That article showed how each could be constructed with the same initial costs, thereby harmonizing that critical aspect of the comparison.
This article further narrows the selection process. The starting point is the supposition that the instrument of choice is a cap contract, leading to the next question: Which cap?
As a starting point, it should be understood that a cap is nothing more than a series of options (caplets), where each caplet protects against the risk of the reset interest rate rising above the caplet’s strike rate as of the start of a given accrual period. Typically, most caps are designed with a common strike rate that applies to all periods covered.
The fact is, caps can be constructed for literally any budget. Choosing a cap with a lower strike rate protects from a lower threshold interest rate and thus would be more expensive. The opposite is true as well: a cap with a higher strike rate protects from a higher threshold rate and is cheaper. Put another way, buying a cheaper cap necessarily foregoes some protection, and vice versa. This tradeoff notwithstanding, it’s not unusual for many entities to make the judgment that all caps are simply too expensive—that the protection received is inadequate or inappropriate, relative to the premium paid. This judgment, however, deserves further consideration.
It’s understandable that anyone purchasing a cap may have some ambivalence. On one hand, given that the cap provides the security of knowing that funding costs will be constrained to some maximum cost of funds, the hedging entity should prefer that the risk being hedged fails to materialize, such that funding will occur more cheaply than that maximum. On the other hand, if the cap/caplet is not constraining, that means that the cap/caplet fails to pay off, and thus the cap/caplet would end up fostering what might seem to be an unnecessary cost. On some level, the company that buys the cap is doing so expecting at least some of the caplets to pay off, i.e., expecting the cap to constrain the effective cost of funds in at least some periods.
Complicating the analysis is the fact that when caps are constructed with a common strike rate for each accrual period, the various caplets that make up the cap will have different degrees of in-the-money-ness, i.e., different portions of intrinsic value (or lack thereof) in each caplet’s premium. In an upward sloping yield curve (as we have in the current economic environment), the levels of prospective interest rate resets generally rise throughout the term of the cap. Assuming a strike rate at some interim interest rate level, the shorter-dated caplets will be out-of-the-money while the longer dated caplets will be in-the-money. With this configuration, the probability of each caplet generating an ending payoff would typically be higher for each successive caplet.
Alternative Cap Possibilities
For the purposes of this article, the discussion relies on data from the end of the day on August 28, 2014 and considers a variety of 36-month LIBOR caps with strike rates ranging from a high of 1.75 percent to a low of 0.25 percent in increments of 0.25 percent. Table 1 shows the full cap premiums (column 2) as well as the per annum premiums (column 3), i.e., the full premium divided by the years covered by the cap. As the strike rate on the cap refers to an annualized interest rate, we can add the per annum premium to the cap’s strike price to generate each cap’s predicted value for the worst case cost of funds that would result in any month with that cap serving as the hedging derivative (column 4).
Table 1: Alternative Cap Valuations (Percent of Notional)
Importantly, this approach reflects an economic orientation that won’t be consistent with the actual cost of funds that would be reported in the firm’s income statement. In the above representation of the worst case outcome, the cap’s premium is allocated ratably across all of the accrual periods covered by the cap. This treatment, however, is not permitted under GAAP. Instead, three alternative, allowable GAAP treatments for caps would allow for three distinct outcomes for when these cap premiums would be reflected in earnings. Those treatments are: hedge accounting where option time value is not excluded from the effectiveness assessment; hedge accounting where option time value is excluded from the effectiveness assessment; or hedge accounting is not applied.
Focusing on the “worst case” outcome may be somewhat of a distraction, in that this result would arise only if every one of the caplets is constraining; but this outcome is highly unlikely in periods of upward sloping yield curves and rising forward rates. In fact, the higher the strike price, the less likely this outcome. For example, if the forward rates as of August 28, 2014 were realized and thus served as the reset interest rates on the assumed variable rate debt, the cap with the 0.25 strike rate would be constraining in 28 of the 36 months being covered, while the cap with the 1.75 strike rate would be constraining in only eight months.
Along with the worst case results, it’s also relevant to assess the more likely outcomes that would arise if the consensus forecast (as reflected by the starting set of forward interest rates) were realized. We can make this assessment by dividing the full premium paid for caps between intrinsic value and time value and recognizing that only time value changes will necessarily be a cost to the hedger. Moreover, the magnitude of this cost is known with certainty from the start of the hedge. In contrast, changes in intrinsic value can be positive or negative. With this segmentation, we can assess the “steady state” case, where the forward rates prevailing at the time the cap is purchased are actually realized. In this situation, all starting intrinsic value would be preserved. The cap would generate a loss, but only in an amount equal to the starting time value.
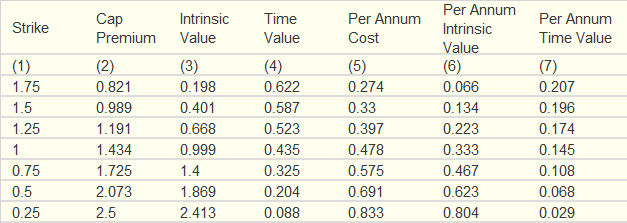
Table 2 shows the same caps as those shown in Tables 1, but in this table the premiums are broken down between their intrinsic and time values. Values in columns 2-4 reflect the amounts for the caps as a whole, and columns 5-7 divide these values by three, to reflect these values on a per annum basis. It’s striking that while the full premiums increase as you move down the rows of the table, such that the amount at risk is rising in each successive row, the respective time values—the costs known with certainty—are actually declining. Put another way, if the reset rates reflected by the configuration of forward interest rates as of the cap’s purchase date are realized, you’d lose more by buying the cheapest of these caps than you would if you were to buy the most expensive.
Table 2: Cap Values (Percent of Notional)

We glean further insight by calculating the expected cost of borrowing under each of these cap hedges, again assuming that starting forward interest rates are realized. These calculations are shown in Table 3. The constrained borrowing rates (column 4) are simply the averages of the starting forward interest rates, constrained by each of the respective caps’ strike rates, and the expected cost of borrowing (column 5) is found by adding the per annum cost of each cap to its associated constrained borrowing rate. Thus, buying the 1.75 percent strike cap assures that funding costs will never exceed 2.024 percent, but the anticipated cost of borrowing would be 1.267 percent—well below that maximum.
Table 3: Expected Cost of Borrowing (Percent, per year)

The last row in this table is also instructive. Too often, the cap’s strike rate is compared with the current short term interest rate, without due consideration of the consensus forecast of where this variable interest rate will likely go over the course of the period being hedged. In this case, the consensus view is that short term rates will be rising over the coming three years, and the unhedged position will likely cost about 1.040 percent per year in interest expenses—again, a value that reflects the average of starting (unconstrained) forward interest rates. Column 6 highlights the marginal impact of each cap relative to that unconstrained average; and column 7 shows the number of months for which the cap is expected to be constraining. The most expensive cap (the 0.25 percent strike cap) increases our expected cost of funds by less than three basis points per year (1.068-1.040 = 0.028, shown in column 6). On the other hand, the cheapest cap (the 1.75 percent strike cap) would raise our expected cost of funds by slightly less than 23 basis points.
Clearly, the more modest the incremental expected cost, the more attractive the cap. But we can’t forget that as attractive as these more expensive caps might be by this analysis, buying caps with lower strike prices (deeper in-the-money caplets) risks bearing a higher cost if the risk being hedged fails to materialize. In assessing this trade-off, though, it’s relevant to consider the respective magnitudes of prospective rate changes from the starting set of forward rates. How much higher—or lower—could those forward rates go during the horizon of the hedge? All else being equal, the expectation that those forward rates are likely to rise should favor buying lower strike caps, and vice versa. But the ultimate choice still requires making a business judgment.
In my own experience dealing with clients in need of hedging instruments, too many take the dismissive attitude that caps are too expensive. I hope reading this article challenges that perspective.
HedgeStar Media Contact Megan Roth, Marketing Manager Office: 952-746-6056 Email: mroth@hedgestar.com
Risk Management Consulting | Valuations Services | Software Advisory | Hedge Accounting Services | Financial Litigation Support

