Switching Caps For Swaps
Publication: AFP Exchange
Caps are an overlooked and less expensive alternative to interest rate swaps
Interest rate swaps are overwhelmingly the instrument of choice for treasury managers who use derivatives for managing their interest risk. For many, though, this preference may be short-sighted, particularly in today’s environment when the yield curve is upward-sloping. Don’t get me wrong: I love interest rate swaps. I just think there are other tools that deserve consideration, as well.
For most non-financial companies, swaps are used in connection with their efforts to manage variable rate debt—most typically, LIBOR-based bank debt or possibly variable rate notes. In these instances, swaps are used to synthetically convert this variable-rate debt to fixed-rate debt, thereby eliminating the exposure to rising interest rates throughout the term (or tenor) of the swap.
Consider the company with a revolver loan tied to three month LIBOR. Clearly, the firm is exposed to the risk of rising interest rates. Suppose the firm expected the balance on this funding to exceed $10 million for the next five years, making it reasonable to consider entering into a five-year, receive variable/pay fixed interest rate swap with a notional size of $10 million. A swap dealer would make a market and quote the required fixed rate on the swap, and the corporate customer would essentially take it or leave it.
For illustrative purposes, we will assume that at the decision point the fixed rate on five-year swaps was 2.35 percent; with all accrual periods, settlement dates, and reset dates and times for this swap identical to those of the loan. With those conditions, the effect of hedging would be to end up with a cost of funds equal to 2.35 percent—plus any spread over LIBOR that the company was obligated to pay on the loan. Entering into the swap requires the execution of an agreement between the counterparties, but generally, all fees and charges are embedded in the stipulated fixed rate of the swap, such that no up-front payment that would be required.
This lack of an initial payment does not exactly mean that the swap is free. It is not. Economists consider the cost of the swap to be an opportunity cost. Effectively the swap serves to lock in an interest rate for the five year horizon—2.35 percent plus the spread. Clearly, if the swap is entered into and LIBOR stays below 2.35 percent, the company would be giving up the benefit of these lower funding costs. Unfortunately, there is no way to know, in advance, whether this opportunity cost will be realized, and if so, how much it would be until it is too late.
Another way to consider the cost of the swap is to measure the “payup cost.” Entering into the swap means that the hedger is effectively extending out to a longer maturity on the yield curve. The current LIBOR value (approximately 30 basis points) applies to a three-month maturity. In an upward sloping yield curve, interest rates are higher for longer maturities. The 2.35 percent fixed rate on the five year swap is the corresponding cost for the five-year horizon. You do not get to lock in 30 basis points for five years; you get to lock in 2.35 percent for five years (in both cases, plus the spread). Thus, we might see the pay-up cost of locking in the fixed rate as being the differential between 2.35 percent and 0.30 percent, or 2.05 percent.
Figure 1 shows the valuation of the at-market five-year swap. It assumes a notional size of $10 million and a fixed rate of 2.35 percent. The receive payments reflect the forward rates shown in the Reset Rate column. Note that the pay payments vary slightly from period to period. This variance arises as a function of the variability of days in the quarter. (The presentation rounds many of the values in the table, so some of the values shown may be difficult to validate.) Multiplying each expected net payment by its associated discount factor gives the present value of each expected cash flow, such that the value of the swap is found by summing these rows. For any at-market swap, these present values sum to zero.

Figure 2 holds all reset interest rate constant at the original LIBOR (0.327 percent). The same notional and fixed rates as those of the at-market swap apply. The value of this swap—slightly less than $1 million—can be thought of as reflecting the cost of moving out on the yield curve and locking in the at-market swap’s fixed rate.
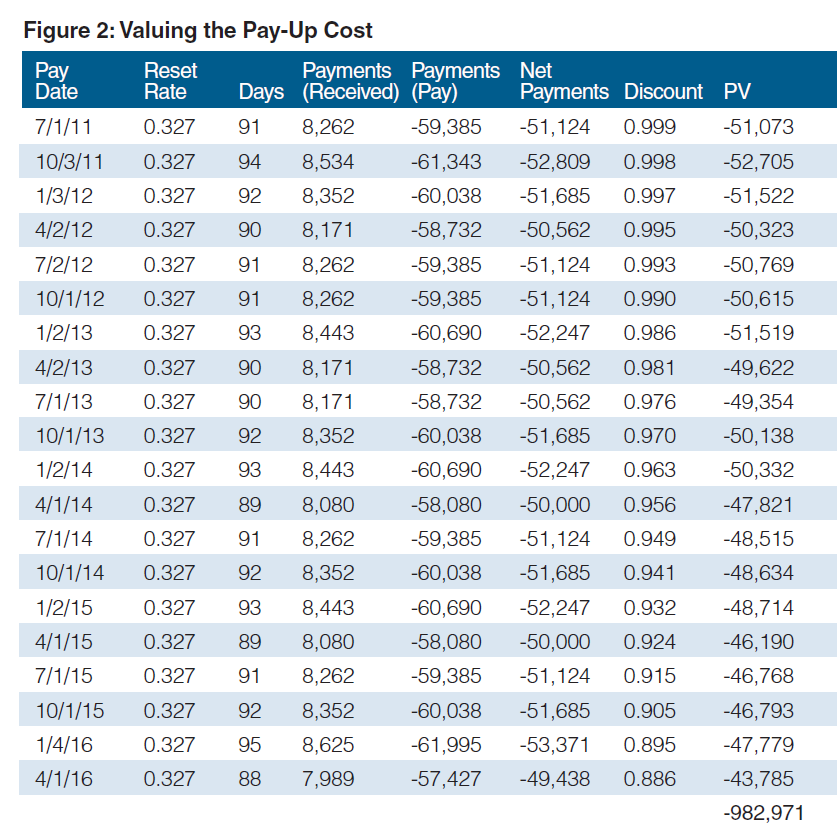
Thus, under this metric, the cost of hedging is $983,000 dollars or 9.83 percent of the notional exposure. Rather than committing to that cost by entering into the swap, suppose we considered buying an interest rate cap for the same money. The cap has the advantage of offering one-sided protection—covering the risk of rising interest rates but allowing the company to benefit from the contingency of lower interest rates. Ultimately our question is this: If we were to pay the pay-up cost as an up front cap premium, what would the terms of that cap be? Figure 3 provides the answer to this question.
Note that the Reset Rates in this exhibit are identical to those of Exhibit 1, i.e., the same forward rates underlying swap valuations apply for cap valuations. Volatilities are market driven, thus given these volatilities and assuming a premium of (approximately) 9.83 percent (reflected by a present value of $983,000), the model derives the cap strike price, which in this case turns out to be 0.343. That is, paying the premium of $983,000 protects us from the prospect of LIBOR rising above 0.343 percent, but it allows us to enjoy exposure to LIBOR when it falls below that threshold.
Admittedly, with LIBOR at 0.327 percent, the prospect of any significant drop in LIBOR is limited. In this example, the worst-case situation would arise when LIBOR reaches or exceeds the cap strike of 0.343 percent.

The effective cost must recognize the cost of the caplet, however. This value is presented as a dollar value in the PV column, or as an annualized yield in the next column (Marginal Cost). Adding the cap strike and this marginal cost yields the worst case cost of funds, period by period in the far right hand column. Note however, that the average of the worst case cost of funds ends up at 2.31 percent (plus any spread). Again, this outcome is the worst case result. It compares favorably to the 2.35 percent that would be locked in, had the swap been selected as the hedging instrument. It also compares favorably with the average of the forward reset rates, which are the market’s consensus forecast of future LIBORs, derived from the currently observed yield curve. From both perspectives, the cap turns out to be an attractive alternative.
Critically, the near-equivalence of the cap’s worst-case outcome to those of the swap’s outcome or that of the consensus unhedged forecast should not be expected to hold all the time. With higher implied volatilities, the caps will be more expensive, and vice versa, so an analysis is required at each decision point, but the lesson here is that caps should not be ignored just because they require an up-front payment. In upward sloping yield curves, liability hedgers will “pay up” to secure a fixed funding rate. Without undertaking the analysis, it is not clear which vehicle would offer the preferred outcome.
Caps protect against the risk of interest rates rising above the cap's strike yield, while leaving the buyer in position to enjoy the benefit of lower rates.
Several other considerations deserve mention:
One:
In all likelihood, institutional hedgers would likely intend to apply hedge accounting; and unfortunately, the hedge accounting rules for options create a disincentive. Under current guidance, the cost of the option is not allocated uniformly over the hedge horizon. Rather, the cost each quarter reflects that quarter’s caplet value. Thus, even though the worst case cost of funds would average 2.31 percent (plus the spread), in fact, the reported costs on the firm’s income statement would generally be rising—sharply—over time. That is a bummer, unless you are planning an imminent exit from the company.
Two:
The analysis presented above reflects an approach that sought to buy an option having a comparable cost to that of the swap’s pay-up cost, but the hedger could elect to spend less. A lower expense could be achieved by either buying a cap with a higher strike or hedging a smaller portion of the exposure. In either case, the hedger would need to appreciate that this decision involves a tradeoff: The lower cost comes with an associated higher risk exposure. Nothing is free.
Three:
An alternative way to address the cost issue, and particularly the sharp rise in the quarterly expenses associated with hedge accounting for caps, would be to enter into a cap with an amortizing notional amount. This is a variant of the decision to hedge only a portion of the exposure. By hedging a declining portion, the steepness of the cost increases would be mitigated. This tactic may likely have particular appeal to the hedger who anticipates phasing into hedges over time. In this way, the effective cost of protection in any given quarter would reflect the average cost of the sequential component caplets that are assembled over the horizon when these.
An interest rate swap serves to eliminate both risk and opportunity. In contrast, an interest rate cap serves to protect against the risk of interest rates rising above the cap’s strike yield, while at the same time leaving the buyer in position to enjoy the benefit of lower rates. Conceptually, on that basis, the caps have to be considered to be the better vehicle—but only if we ignore costs.
Unfortunately, most hedgers who potentially could use either tool tend to assess costs inappropriately: They compare the respective initial cash flows—nothing for the swap, versus the cap’s initial premium—and conclude that caps are too expensive. This comparison, however, is flawed. The better comparison would be to compare the cap’s initial cost to the pay-up cost of the swap. On that basis, caps are going to start to appear to be way more attractive.
HedgeStar Media Contact
Megan Roth, Marketing Manager
Office: 952-746-6056
Email: mroth@hedgestar.com

